Wie berechne ich das resultierende Moment, in einem bestimmten Punkt?

Question

Auf einen Quader greifen die Kräfte F1, F2 und F3 in den Punkten A, B und C an. Berechnen Sie die resultierende Kraft R und das resultierende Moment M_{Dreh} der drei Kräfte bezüglich des Punktes D.

$$ r_A = \begin{pmatrix} 0\\0\\0 \end{pmatrix} r_B = \begin{pmatrix} 0\\2α\\α \end{pmatrix} r_C = \begin{pmatrix} 4α\\2α\\0 \end{pmatrix} r_D = \begin{pmatrix} 2α\\α\\α \end{pmatrix} F_1 = F\begin{pmatrix} 1\\0\\1 \end{pmatrix} F_2 = F\begin{pmatrix} 0\\-1\\-1 \end{pmatrix} F_3 =F \begin{pmatrix} -1\\-1\\0 \end{pmatrix}$$

Kann mir vielleicht jemand sagen, wie ich diese Aufgabe lösen kann? Ich soll die resultierende Kraft berechnen und das resultierende Moment , im Punkt D. Die resultierende Kraft habe ich mittels Vektor addition zwischen den Kräften F1-F3 errechnet. Ich habe bei F(0,-2,0) raus. Wie berechne ich hier das Moment? Muss ich um den Punkt D drehen und dann die einzelnen Kräfte mitnehmen, oder wie gehe ich hier am besten vor?

Es sind verschiedene Richtungsvektoren gegeben und Kräfte, die Kräfte bzw. das Resultat habe ich der Kräfte bereits ausgerechnet. Mir fehlt nur noch das Resultierende Moment.

Über jede Hilfe bin ich dankbar.

Answer 1

Hallo

bestimme zuerst die 3 Vektoren r die von D zu den Angriffspunkten der Kräfte gehen.

 dann gilt M=ri x Fi mit x Vektorprodukt. M, r, F Vektoren. am Ende die 3 Drehmomente addieren.

Gruß lul

Comments

Könntest du mit mir vielleicht beim ersten Vektor auf die Sprünge helfen, weil ich echt keine Ahnung habe, wie ich die Aufgabe lösen kann.

Wenn ich jetzt beispielsweise R1 betrachte (0;0;0), diesen kann ich ja schlecht einzeichnen. Bei R2(0;2;1). Der würde ja theoretisch genau da liegen, wo F1 liegt oder? Und wie gehe ich nun vor? Ein Beispiel für den ersten Vektor würde mir reichen, um es zu verstehen.

Answer 2

Hallo Marco,

ich unterstelle, dass Dir das Kreuz- (bzw. Vektor-)Produkt fremd ist. Ich habe dies schon mal in dieser Antwort näher erklärt. Du benötigst dazu den Hebelarm - d.h. den Vektor von \(D\) zum Angriffspunkt der Kraft und die Kraft selbst.

Der Vektor \(\vec{r}_D\) zum Punkt \(D\) ist

$$\vec{r}_D = \begin{pmatrix} 2a\\ a\\ a\end{pmatrix}$$ Der Hebelarm \(\vec{DB}\) von \(D\) nach z.B. \(B\) ist

$$\vec{DB} = \vec{r}_B - \vec{r}_D = \begin{pmatrix} 0\\ 2a\\ a\end{pmatrix} - \begin{pmatrix} 2a\\ a\\ a\end{pmatrix} = \begin{pmatrix} -2a\\ a\\ 0\end{pmatrix}$$ prüfe dies in der Skizze noch mal nach! Du muss von \(D\) die Strecke \(2a\) in X-Richtung zurück (negativ) und dann um \(a\) in positive Y-Richtung um nach \(B\)  zu kommen. Die Kraft \(F_2\) selbst, die in \(B\) angreift, ist bereits gegeben. Dann ist das Moment \(M_{D2}\) (Kreuzprodukt) welches durch \(F_2\) um den Punkt \(D\) erzeugt wird:

$$\begin{aligned}M_{D2} &= \vec{DB} \times F_2 = \begin{pmatrix} -2a\\ a\\ 0\end{pmatrix} \times \begin{pmatrix} 0\\ -1\\ -1\end{pmatrix}F \\ &= \begin{pmatrix} a \cdot (-1) - 0 \cdot (-1)\\ 0 \cdot 0 - (-2a)\cdot(-1)\\ (-2a)\cdot(-1) - a \cdot 0\end{pmatrix}F= \begin{pmatrix} -1\\ -2\\ 2\end{pmatrix}aF\end{aligned}$$

die Momente, die durch \(F_1\) und \(F_3\) eingebracht werden, lassen sich genauso berechnen. Zur Kontrolle - die Summe \(M_D\) aller Momente um \(D\) ist:

$$M_D= \begin{pmatrix} -3\\ 0\\ 2\end{pmatrix}aF$$ Gruß Werner

PS.: Die ähnliche Frage Wie kann man das resultierende Moment berechnen? trifft Dein Problem ziemlich gut.

Comments

Danke dir für deine Antwort. Ich habe das gleiche Prinzip angewendet und folgendes für MD1 raus=(-1+3-1)

MD2=(-1-2+2)

MD3=(0,0,-3) raus. Wie kann ich diese miteinander verrechnen, damit ich MD rausbekomme, sowie du es rausbekommen hast?

Ich habe versucht MD1 sowie MD2 miteinander zu berrechnen, also mit dem Kreuzprodukt, das Ergebnis mit MD3 Kreuzprodukt, leider nicht das selbe, wie du erhalten, ich habe für MD=(-3,+12,0) raus. Kann es sein, das ich die berechneten Werte MD1,MD2,MD3 mittels anderer Rechenoperation berechne? Oder muss ich hier die ra-rd Vektoren ansehen und mit denen was machen? Ich habe mir den Link angeschaut, aber konnte leider nicht ganz nachvollziehen was ich machen soll.

PS: ist dann das MD, auch gleich MDres?

---

Hat sich geklärt, trotzdem danke! Hatte einen Zahlendreher. Habe allerdings für MD=(-3,0-2) raus. Falls du das noch mal nachprüfen könntest, wäre ich dir trotzdem sehr dankbar.

Mir wäre es noch trotzdem wichtig zu wissen, ob MD auch gleich MDres darstellt? Weil in der Aufgabnenstellung. Danach gefragt worden ist, also. Brauche ich nicht MD1-MD3 aufschreiben?

---

Meine Ergebnisse sind:

$$\begin{aligned}M_{D1} &= \begin{pmatrix} -1\\1\\1 \end{pmatrix}aF \\ M_{D2}&= \begin{pmatrix} -1\\-2\\2 \end{pmatrix}aF \\ M_{D3}&=\begin{pmatrix} -1\\1\\-1 \end{pmatrix}aF \end{aligned}$$

Und das \(M_D\) - (bzw. \(M_{D\text{res}}\); das ist das gleiche!) - ist die Summe dieser drei Momente. Hier nochmal die Berechnung von \(M_{D1}\):

$$\begin{aligned} M_{D1} &= (\vec{r}_A - \vec{r}_D) \times F_1 \\& = \left( \begin{pmatrix} 0\\0\\0 \end{pmatrix} - \begin{pmatrix} 2a\\a\\a \end{pmatrix}\right) \times \begin{pmatrix} 1\\0\\1 \end{pmatrix}F \\ &= \begin{pmatrix} -2\\ \colorbox{#ffff44}{-1} \\ \colorbox{#88ccff}{-1} \end{pmatrix} \times \begin{pmatrix} \colorbox{#88ff88}{1}\\0\\ \colorbox{#ffcc88}{1} \end{pmatrix}aF \\ &= \begin{pmatrix} \colorbox{#ffff44}{-1} \cdot \colorbox{#ffcc88}{1} - (\colorbox{#88ccff}{-1}) \cdot 0\\ \colorbox{#88ccff}{-1} \cdot \colorbox{#88ff88}{1} - (-2) \cdot \colorbox{#ffcc88}{1}\\-2 \cdot 0 - (\colorbox{#ffff44}{-1}) \cdot \colorbox{#88ff88}{1} \end{pmatrix}aF\\ &= \begin{pmatrix} -1\\1\\1 \end{pmatrix}aF \end{aligned}$$

Ich habe die einzelnen Werte in den Vektoren teilweise farblich markiert, so sieht man welche 1 bzw. -1 wo hinkommt.

Und für \(M_{D3}\) habe ich Dir das grafisch im Geoknecht3D eingegeben. Also nur \(F_3\) und das resultierende Moment um \(D\).

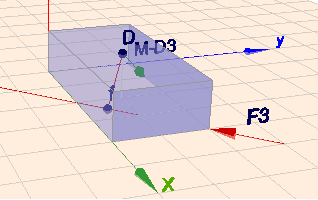
(klick auf das Bild und bewege die Szene mit der Maus)

Du siehst dort die Kraft \(F_3\) und ihre Wirklinie (rot). Vom Punkt \(D\) habe ich das Lot auf die Wirklinie gefällt. Dort erhalte ich den Punkt \(f\). Die Strecke \(Df\) ist der Hebelarm für \(F_3\) um den Punkt \(D\). Das Moment steht senkrecht auf dem Hebelarm und senkrecht auf der Wirklinie. Also rein optisch ist das Ergebnis (s.o.) ok!

Mir wäre es noch trotzdem wichtig zu wissen, ob MD auch gleich MDres darstellt?

Ja - das ist so (s.o.). Es ist die Summe aller Momente.

Brauche ich nicht MD1-MD3 aufschreiben?

das verstehe ich nicht? Ziehst Du die beiden Momente von einander ab?

Gruß Werner

---

Vielen Dank!

Nach dem ich das noch mal augerechnet have, habe ich das selbe raus!

Letzte frage : wenn ich beispielsweise md1 berechne, und dann den kreuz Produkt zwischen DB X F2 mache, wäre es falsch wenn ich es anders rum mache, also F2 X DB? Muss ich hier etwas beachten, ich habe das beispielsweise so gemacht und andere Werte erhalten. Oder müsste hier das selbe rauskommen?

Gruß Marko

---

Hallo Marko,

wenn ich beispielsweise md1 berechne, und dann den kreuz Produkt zwischen DB X F2 mache, wäre es falsch wenn ich es anders rum mache, also F2 X DB?

ja - es wäre falsch. Das Ergebnis wäre dann das negative Moment. Der Zusammenhang ist: $$\vec{DB} \times F_2 = - (F_2 \times \vec{DB})$$ Gruß Werner

---

Ich muss aber allerdings, meine Momente, die ich in jedem Punkt bestimmt habe, in aller Fälle addieren und nicht subtrahieren, wenn ich das resultierende Moment haben möchte?

---

Ja natürlich. Was anderes macht physikalisch auch keinen Sinn. Wie kommst Du darauf, dass sie subtrahiert werden müssen?