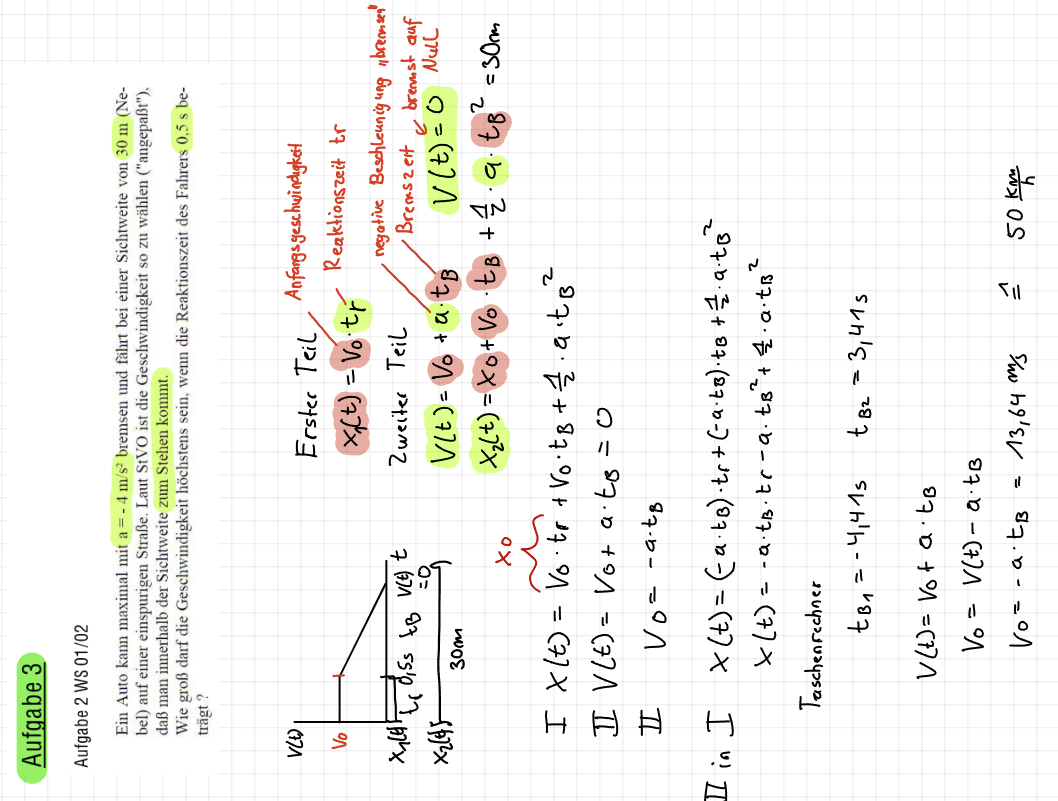
Text erkannt:
Aufgabe 3
Aufgabe 2 WS 01/02
Ein Auto kann maximal mit \( \mathrm{a}=-4 \mathrm{~m} / \mathrm{s}^{2} \) bremsen und fährt bei einer Sichtweite von \( 30 \mathrm{~m} \) (Nebel) auf einer einspurigen Straße. Laut StVO ist die Geschwindigkeit so zu wählen ("angepaßt"), daß man innerhalb der Sichtweite zum Stehen kommt.
Wie groß darf die Geschwindigkeit höchstens sein, wenn die Reaktionszeit des Fahrers \( 0,5 \mathrm{~s} \) beträgt?
\( I x(t)=\overbrace{V_{0} \cdot t_{1}}^{x_{0}}+V_{0} \cdot t_{B}+\frac{1}{2} \cdot a \cdot t_{B}{ }^{2} \)
II \( V(t)=V_{O}+a \cdot t_{B}=0 \)
II \( V_{O}=-a \cdot t_{B} \)
\( \text { II in I } \begin{aligned} x(t) &=\left(-a \cdot t_{B}\right) \cdot t_{C}+\left(-a \cdot t_{B}\right) \cdot t_{B}+\frac{1}{2} \cdot a \cdot t_{B}^{2} \\ x(t) &=-a \cdot t_{B} \cdot t_{C}-a \cdot t_{B}^{2}+\frac{1}{2} \cdot a \cdot t_{B}{ }^{2} \end{aligned} \)
Taschenrechner
\( t_{B_{1}}=-4,41 \mathrm{~s} \quad t_{B_{2}}=3,41 \mathrm{~s} \)
\( V(t)=V_{0}+a \cdot t_{B} \)
\( V_{0}=V(t)-a \cdot t_{B} \)
\( V_{0}=-a \cdot t_{B}=13,64 \mathrm{~m} / \mathrm{s} \cong \triangleq 0 \frac{\mathrm{km}}{\mathrm{h}} \)
Hi, ich verstehe überhaupt nicht was ich da in den Taschenrechner eingeben muss? Da ich tb nicht habe... Die Lösung ist von nem Tutor von mir.